· Prime Limit
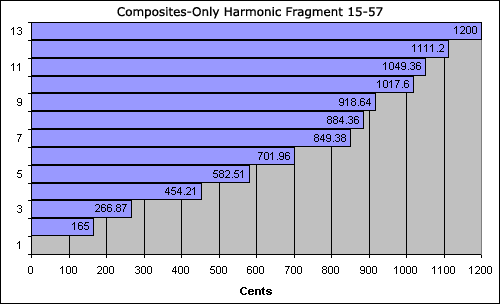
· Octave RepeatingThis tuning also has the lowest harmonic complexity in this series of composites only tunings. As you might expect, with its starting composite of 3x5, it also has the most common tones. The degree corresponding to harmonic 49 is the only one that doesn't have a factor in common with other tones in the scale. However, it's still a very violent tuning, with a very strong curvature of the spine, so to speak.
A red number in the Rounded Tuning Table column flags it as beyond the +/-99 cent limit set by some hardware synthesizer tuning tables.
| Scale Degree | Har- monic | Ratio | Quotient | Cents | Tuning Table | Rounded Tuning Table | Note Name |
|---|---|---|---|---|---|---|---|
| 1 | 15 | 1:1 | 1 | 0.00 | -116.76 | -117 | C |
| 2 | 33 | 11:10 | 1.1 | 165.00 | -51.75 | -52 | C# |
| 3 | 35 | 7:6 | 1.166666667 | 266.87 | -49.89 | -50 | D |
| 4 | 39 | 13:10 | 1.3 | 454.21 | 37.46 | 37 | D# |
| 5 | 21 | 7:5 | 1.4 | 582.51 | 65.75 | 66 | E |
| 6 | 45 | 3:2 | 1.5 | 701.96 | 85.20 | 85 | F |
| 7 | 49 | 49:30 | 1.633333333 | 849.38 | 132.62 | 133 | F# |
| 8 | 25 | 5:3 | 1.666666667 | 884.36 | 67.60 | 68 | G |
| 9 | 51 | 17:10 | 1.7 | 918.64 | 1.88 | 2 | G# |
| 10 | 27 | 9:5 | 1.8 | 1017.60 | 0.84 | 1 | A |
| 11 | 55 | 11:6 | 1.833333333 | 1049.36 | -67.40 | -67 | A# |
| 12 | 57 | 19:10 | 1.9 | 1111.20 | -105.56 | -106 | B |
Play Tuning
| Largest step size: | 187.34 c | (39:35) |
| Smallest step size: | 31.77 c | (55:54) |
| Number of step sizes: | 12 | |
| Prime Limit: | 19 | |
| Odd Limit: | 49 | |
| HC = | 25.8 | |
2005 by David J. Finnamore
tuning@elvenminstrel.com